Diseño y validación de un instrumento para observación de clases de matemáticas en Educación Secundaria: grupo nominal y método Delphi

Blanca Arteaga-Martínez; Jesús Macías-Sánchez; Marta Pla-Castells; Mónica  Ramírez-García

doi:10.30827/relieve.v27i2.21812

作者

Blanca Arteaga-Martínez Universidad Nacional de Educación a Distancia (UNED)
Jesús Macías-Sánchez Universidad Complutense de Madrid
Marta Pla-Castells Universitat de València
Mónica Ramírez-García Centro Universitario La Salle

##plugins.pubIds.doi.readerDisplayName##:

https://doi.org/10.30827/relieve.v27i2.21812

关键词:

德尔菲法, 名义群体法, 数学教学, 中等教育, 观察工具

摘要

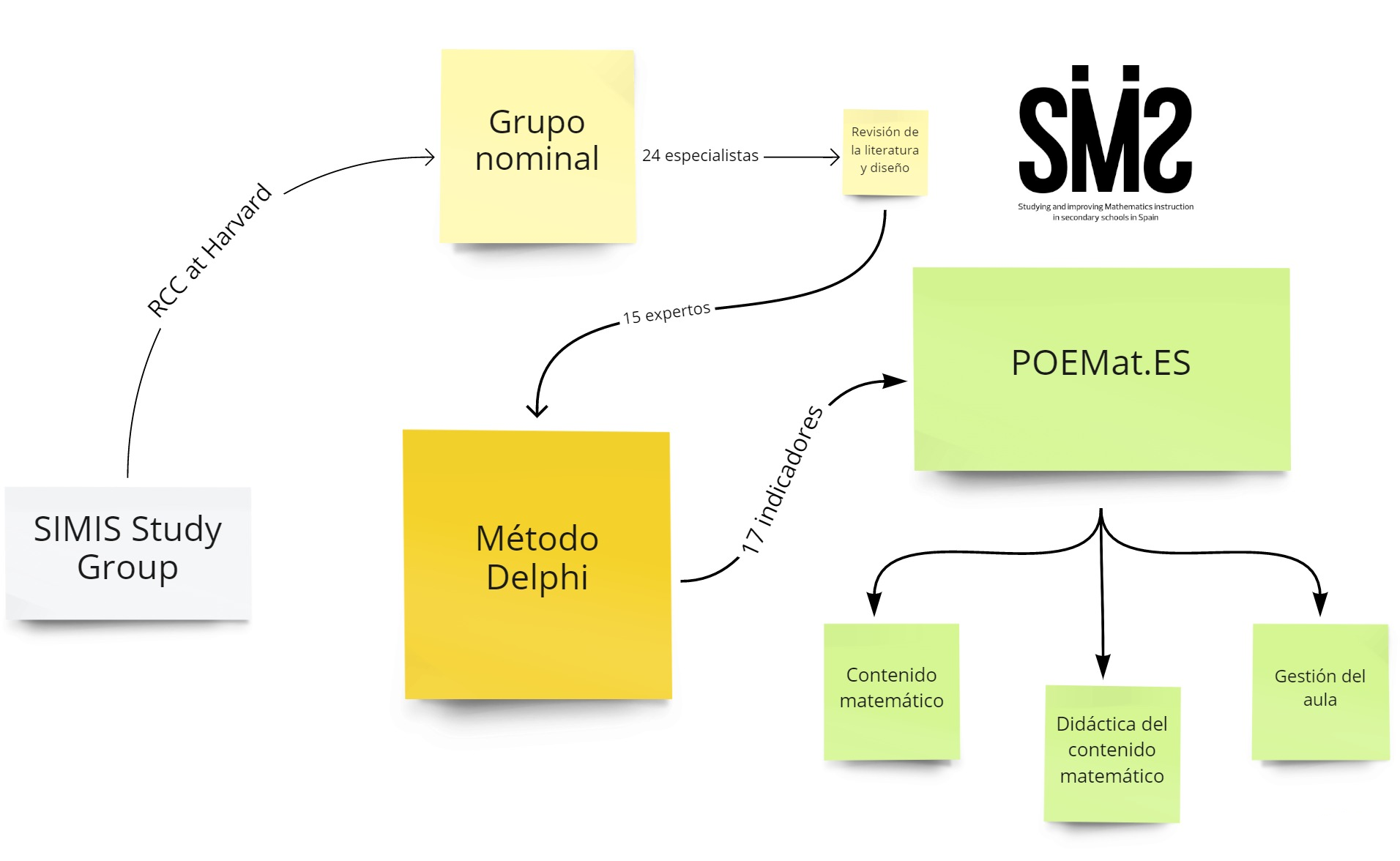
在西班牙，人们不常将教学实践观察及阐释作为教师学习的工具。为了促进它在实践中的应用，拥有一款既能简化分析过程，又能同时收集其应用教学领域特性的工具至关重要。因此该研究旨在呈现POEMat.ES的设计、建设及验证过程。POEMat.ES是一款对中等教育数学课堂教师实践进行观察及阐释的工具。研究分为两个阶段：（1）由24名专家组成的名义群体对文献及设计进行审校；（2）关于工具的建设及验证，使用德尔菲法对15名专家进行两轮的意见征询。工具最终版本由三个维度组成：数学内容、相应内容的教学法和课堂管理。在三个维度的基础上细分为17个指标，每个指标涵盖四个等级。一致认为研究使用的共识方法适用于选择及验证教师实践观察的指标。该研究最终证实，在教师实践观察系统化及对西班牙中等教育数学教学过程的准确解读上，POEMat.ES都是一款有效的工具。

##plugins.generic.usageStats.downloads##

##plugins.generic.usageStats.noStats##

##submission.authorBiographies##

##submission.authorWithAffiliation##

Licenciada en CC. Matemáticas por la Universidad Autónoma de Madrid y Doctora en CC. de la Educación por la Universidad Complutense de Madrid. Ha combinado su labor como profesora en niveles obligatorios con la docencia en la universidad (Universidad Carlos III de Madrid, Saint Louis University in Madrid, Universidad Internacional de La Rioja, Universidad de Alcalá y Universidad Rey Juan Carlos), además de desarrollar labores de coordinación de equipos. En la actualidad profesora Ayudante Doctora en la Universidad Nacional de Educación a Distancia (UNED). Su investigación se ha centrado en la investigación-acción en el aula, tomando la didáctica de la matemática como eje vertebrador. Ha dirigido y participado en distintos proyectos de investigación subvencionados y proyectos de innovación educativa con distintas universidades. Integrante del grupo de investigación Pedagogía Adaptativa de la Universidad Complutense de Madrid.

##submission.authorWithAffiliation##

Licenciado en CC. Matemáticas por la Universidad Complutense de Madrid, Máster en Formación del profesorado y Máster en estudios Avanzados en Pedagogía por la Universidad Complutense de Madrid y Doctor en CC. de la Educación por la Universidad Complutense de Madrid. Combina su labor como profesor en niveles obligatorios con la docencia en la universidad (Universidad Internacional de La Rioja, Universidad Europea de Madrid y Universidad Complutense de Madrid). En la actualidad profesor Asociado en la Universidad Complutense de Madrid (UCM) y profesor en Enseñanza Obligatoria en el Colegio Brotmadrid. Su investigación se ha centrado en la investigación-acción en el aula, fundamentado en el proceso de enseñanza-aprendizaje de las matemáticas Ha participado en algún proyecto de investigación subvencionado y proyectos de innovación educativa.

##submission.authorWithAffiliation##

Licenciada en CC. Matemáticas por la Universitat de València (UVEG) y Doctora en CC. de la Computación por la misma universidad. Ha trabajado como directora de proyectos europeos para el Instituto de Robótica y TIC de la UVEG mientras era profesora asociada en departamentos de la Facultad de Matemáticas de la UVEG y de la Universitat Jaume I de Castelló (UJI). En la actualidad es profesora contratada doctora en la UVEG. Por su experiencia en programación de software, ha sido capaz aplicar las nuevas tecnologías en el campo de la educación matemática. Ha trabajado también en formación continua de profesores de primaria en colaboración con el Centro de formación, innovación y recursos para el profesorado de la Generalitat Valenciana. En la actualidad pertenece al grupo de investigación de Modelización Matemática del departamento de Didáctica de la Matemática de la UVEG.

##submission.authorWithAffiliation##

Licenciada en CC. Matemáticas por la Universidad Autónoma de Madrid y Doctora en CC. de la Educación por la Universidad Complutense de Madrid. Trabaja como profesora asociada en la Unidad de Didáctica de las Matemáticas de la Universidad Complutense de Madrid desde 2011 y como profesora titular en el Centro Universitario de La Salle desde 2006. Su investigación se ha centrado en la enseñanza-aprendizaje de las matemáticas en las primeras edades. Desde 2016 colabora con varios investigadores de la Universidad de Sevilla y de la Universidad de Huelva aplicando el modelo de conocimiento especializado del profesor de matemáticas (MTSK) a su actividad como formadora de maestros (especialmente de Educación Infantil en formación inicial y formación permanente) y como investigadora.

参考

Aguilar-González, A., Muñoz-Catalán, M.C., Carrillo-Yáñez, J., & Rodríguez-Muñiz, L. J. (2018). ¿Cómo establecer relaciones entre conocimiento especializado y concepciones del profesorado de matemáticas? PNA, 13(1), 41-61. http://dx.doi.org/10.30827/pna.v13i1.7944

Barriendos, A., Berger, B., Domínguez, E., & Martínez, M. V. (2018). Manual PROMATE. Pauta de observación de clases de matemáticas impartidas por profesores principiantes. Centro de Investigación Avanzada en Educación de Chile.

Bostic, J., Lesseig, K., Sherman, M., & Boston, M. (2021). Classroom observation and mathematics education research. Journal of Mathematics Teacher Education, 24, 5–31. https://doi.org/10.1007/s10857-019-09445-0

Boston, M.D., & Candela, A.G. (2018). The Instructional Quality Assessment as a tool for reflecting on instructional practice. ZDM Mathematics Education, 50(3), 427-444. https://doi.org/10.1007/s11858-018-0916-6

Brousseau, G. (2007). Iniciación al estudio de la teoría de las situaciones didácticas. Libros del Zorzal.

Buck, A. J., Gross, M., Hakim, S., & Weinblatt, J. (1993). Using the Delphi process to analyze social policy implementation: A post hoc case from vocational rehabilitation. Policy Sciences, 26, 271-288. https://doi.org/10.1007/BF00999473

Cabero, J. (2014). Formación del profesorado universitario en TIC. Aplicación del método Delphi para la selección de los contenidos formativos. Educación XX1, 17(1), 111-132. https://doi.org/10.5944/educxx1.17.1.10707

Cabero, J., & Barroso, J. (2013). La utilización del juicio de experto para la evaluación de TIC: el coeficiente de competencia experta. Bordón. Revista de Pedagogía, 65(2), 25-38. https://doi.org/10.13042/brp.2013.65202

Cabero, J., & Infante, A. (2014). Empleo del método Delphi y su empleo en la investigación en comunicación y educación. EDUTEC. Revista electrónica de tecnología educativa, 48, a272-a272. https://doi.org/10.21556/edutec.2014.48.187

Cabero, J., & Llorente, M. C. (2013). La aplicación del juicio de experto como técnica de evaluación de las tecnologías de la información y comunicación (TIC). Revista de Tecnología de Información y Comunicación en Educación, 7(2), 11-22.

Charalambous, C.Y., & Praetorius, A.K. (2018). Studying mathematics instruction through different lenses: setting the ground for understanding instructional quality more comprehensively. ZDM Mathematics Education, 50(3), 355–366. https://doi.org/10.1007/s11858-018-0914-8

Clayton, M. J. (1997). Delphi: a technique to harness expert opinion for critical decision‐making tasks in education. Educational psychology, 17(4), 373-386. https://doi.org/10.1080/0144341970170401

Diego-Mantecón, J. M., & Córdoba-Gómez, F. J. (2019). Adaptación y validación del MRBQ (Mathematics-Related Beliefs Questionnaire) al contexto colombiano con estudiantes de secundaria. Educación matemática, 31(1), 66-91. https://doi.org/10.24844/em3101.03

Dolores, C., & Ibáñez, G. (2020). Conceptualizaciones de la Pendiente en Libros de Texto de Matemáticas. Bolema: Boletim de Educação Matemática, 34, 825-846. https://doi.org/10.1590/1980-4415v34n67a22

Duval, R. (1993). Registros de representación semiótica y funcionamiento cognitivo del pensamiento. En E. Hitt (Ed.), Investigaciones en Matemática Educativa II (pp. 173-201). Grupo Editorial Iberoamérica.

Eccius-Wellmann, C., Lara-Barragán, A. G., Martschink, B., & Freitag, S. (2017). Comparación de perfiles de ansiedad matemática entre estudiantes mexicanos y estudiantes alemanes. Revista iberoamericana de educación superior, 8(23), 69-83. https://doi.org/10.22201/iisue.20072872e.2017.23.246

Garzón, D. (2017). Análisis de las decisiones del profesor de matemáticas en su gestión de aula. Revista Educación Matemática, 29(3), 131-160. https://doi.org/10.24844/EM2903.05

Gordon, T. J. (1994). The Delphi method. In J.C. Glenn & T.J. Gordon (Eds.). Futures Research Methodology Version 2.0. (CD-Rom). American Council for the United Nations University.

Hill, H., Blunk, M.L., Charalambous, C.Y., Lewis, J.M., Phelps, G.C., Sleep, L., & Ball, D. (2008). Mathematical Knowledge for Teaching and the Mathematical Quality of Instruction: An Exploratory Study. Routledge Cognition and Instruction, 26(4). https://doi.org/10.1080/07370000802177235

Kaiser, G. (2020) Mathematical Modelling and Applications in Education. In S. Lerman (Eds.), Encyclopedia of Mathematics Education. Springer. https://doi.org/10.1007/978-3-030-15789-0_101

Landeta, J. (1999). El método Delphi. Una técnica de previsión para la incertidumbre. Ariel.

Linstone, H. A., & Turoff, M. (1975). The delphi method. Addison-Wesley.

Liu, R. D., Wang, J., Star, J. R., Zhen, R., Jiang, R. H., & Fu, X. C. (2018). Turning potential flexibility into flexible performance: Moderating effect of self-efficacy and use of flexible cognition. Frontiers in psychology, 9, 646. https://doi.org/10.3389/fpsyg.2018.00646

Llorente, L. (2013). Assessing Personal Learning Environments (PLEs). An expert evaluation. Journal of New Approaches in Educational Research), 2(1), 39-44. https://doi.org/10.7821/naer.2.1.39-44

López-Gómez, E. (2018). El método Delphi en la investigación actual en educación: una revisión teórica y metodológica. Educación XX1, 21(1), 17-40. https://doi.org/10.5944/educXX1.20169

Ludwig, B. (1997). Predicting the future: Have you considered using the Delphi methodology. Journal of extension, 35(5), 1-4.

Martínez, A. (1988). Diseños experimentales: métodos y elementos de teoría. Trillas.

Martino, J. (1999). Thirty years of change and stability. Technological Forecasting and Social Change, 62(1-2), 13-18. https://doi.org/10.1016/S0040-1625(99)00011-6

Martins, G. A., & Theóphilo, C. R. (2007). Metodologia da Investigação Científica para Ciências Sociais Aplicadas. Atlas

Matsumura, L. C., Garnier, H., Slater, S. C., & Boston, M. (2008). Toward measuring instructional interactions ‘at-scale’. Educational Assessment, 13(4), 267–300. https://doi.org/10.1080/10627190802602541

Maz-Machado, A., Madrid, M. J., León-Mantero, C., & Jiménez-Fanjul, N. (2019). Mathematical practical sessions with manipulatives: Trainee teachers’ perceptions of their utility. South African Journal of Education, 39(2), Art. #1620. https://doi.org/10.15700/saje.v39ns2a1620

McMillan, S.S., King, M., & Tully, M.P. (2016). How to use the nominal group and Delphi techniques. International Journal of Clinic Pharmacy, 38, 655–662. https://doi.org/10.1007/s11096-016-0257-x

Mengual, S., Roig, R., & Mira, J.B. (2016). Delphi study for the design and validation of a questionnaire about digital competences in higher education. International Journal of Educational Technology in High Education, 13, 12. https://doi.org/10.1186/s41239-016-0009-y

Molina, O., & Samper, C. (2019). Tipos de problemas que provocan la generación de argumentos inductivos, abductivos y deductivos. Bolema: Boletim de Educação Matemática, 33, 109-134. https://doi.org/10.1590/1980-4415v33n63a06

Moore, C. M. (1987). Group techniques for idea building. Sage Publications, Inc.

Novakowski, N., & Wellar, B. (2008). Using the Delphi technique in normative planning research: methodological design considerations. Environment and Planning A, 40(6), 1485-1500. https://doi.org/10.1068/a39267

Okoli, C., & Pawlowski, S. D. (2004). The Delphi method as a research tool: an example, design considerations and applications. Information & management, 42(1), 15-29. https://doi.org/10.1016/j.im.2003.11.002

Openshaw, R., & Walshaw, M. (2019). Transnational Synergies in School Mathematics and Science Debates. Springer Nature. https://doi.org/10.1007/978-3-030-28269-1

Orton, A. (2003). Didáctica de las matemáticas. Ministerio de Educación, Cultura y Deporte-Ediciones Morata.

Parenté, F. J., & Anderson, J.K. (1987). Delphi inquiry systems. En G. Wright & P. Ayton (Eds.), Judgemental Forecasting (pp. 129-156). John Wiley.

Patton, M. (1990). Qualitative evaluation and research methods. Newbury Park, CA.

Piburn, M., & Sawada, D. (2000). Reformed teaching observation protocol (RTOP): Reference manual. Technical Report. Arizona Collaborative for Excellence in the Preparation of Teachers.

Planas, N., Arnal-Bailera, A., & García-Honrado, I. (2018). El discurso matemático del profesor: ¿Cómo se produce en clase y cómo se puede investigar? Enseñanza de las Ciencias, 36(1), 45-60. https://doi.org/10.5565/rev/ensciencias.2240

Praetorius, A. K., & Charalambous, C. Y. (2018). Classroom observation frameworks for studying instructional quality: looking back and looking forward. ZDM Mathematics Education, 50(3), 535-553. https://doi.org/10.1007/s11858-018-0946-0

Rodríguez, J.M., & Ruiz, J. (2019). El clima social en centros educativos: percepción del profesorado de Educación Secundaria Obligatoria de la Comunidad de Madrid. Revista de Investigación Educativa, 37(1), 231-250. https://doi.org/10.6018/rie.37.1.320541

Rowland, T., Turner, F., Thwaites, A., & Huckstep, P. (2009). Transformation: Using examples in mathematics teaching. In Developing Primary Mathematics Teaching: Reflecting on Practice with the Knowledge Quartet (pp. 67-100). SAGE Publications. https://doi.org/10.4135/9781446279571

Schlesinger, L., & Jentsch, A. (2016). Theoretical and methodological challenges in measuring instructional quality in mathematics education using classroom observations. ZDM Mathematics Education, 48, 29–40. https://doi.org/10.1007/s11858-016-0765-0

Schneider, M., Rittle-Johnson, B., & Star, J. R. (2011). Relations between conceptual knowledge, procedural knowledge, and procedural flexibility in two samples differing in prior knowledge. Developmental Psychology, 47(6), 1525-1538. https://doi.org/10.1037/a0024997

Shah, H., & Kalaian, S. A. (2009). Which Parametric Statistical Method to Use For Analyzing Delphi Data? Journal of Modern Applied Statistical Method, 8(1), 226-232. https://doi.org/10.22237/jmasm/1241137140

Teddlie, C., Creemers, B., Kyriakides, L., Muijs, D., & Yu, F. (2006). The international system for teacher observation and feedback: Evolution of an international study of teacher effectiveness constructs. Educational Research and Evaluation, 12(6), 561–582. https://doi.org/10.1080/13803610600874067

Thomas, C. A., & Berry III, R. Q. (2019). A Qualitative Metasynthesis of Culturally Relevant Pedagogy & Culturally Responsive Teaching: Unpacking Mathematics Teaching Practices. Journal of Mathematics Education at Teachers College, 10(1), 21-30. https://doi.org/10.7916/jmetc.v10i1.1668

Thompson, C. J., & Davis, S. B. (2014). Classroom observation data and instruction in primary mathematics education: Improving design and rigour. Mathematics Education Research Journal, 26(2), 301–323. https://doi.org/10.1007/s13394-013-0099-y

van den Ham, A. K., & Heinze, A. (2018). Does the textbook matter? Longitudinal effects of textbook choice on primary school students’ achievement in mathematics. Studies in Educational Evaluation, 59, 133-140. https://doi.org/10.1016/j.stueduc.2018.07.005

Van Zoest, L. R., Peterson, B. E., Rougée, A. O., Stockero, S. L., Leatham, K. R., & Freeburn, B. (2021). Conceptualizing important facets of teacher responses to student mathematical thinking. International Journal of Mathematical Education in Science and Technology, 1-26. https://doi.org/10.1080/0020739X.2021.1895341

Vergnaud, G. (2013). Pourquoi la théorie des champs conceptuels? Infancia y Aprendizaje, 36(2), 131-161. https://doi.org/10.1174/021037013806196283

von der Gracht, H.A. (2012). Consensus measurement in Delphi studies: review and implications for future quality assurance. Technological Forecasting and Social Change, 79(8), 1525-1536. https://doi.org/10.1016/j.techfore.2012.04.013

Walkowiak, T. A., Berry, R. Q., Meyer, J. P., Rimm-Kaufman, S. E., & Ottmar, E. R. (2014). Introducing an observational measure of standards-based mathematics teaching practices: Evidence of validity and score reliability. Educational Studies in Mathematics, 85(1), 109–128. https://doi.org/10.1007/s10649-013-9499-x

Weber, K. E., Gold, B., Prilop, C. N., & Kleinknecht, M. (2018). Promoting pre-service teachers' professional vision of classroom management during practical school training: Effects of a structured online-and video-based self-reflection and feedback intervention. Teaching and Teacher Education, 76, 39-49. https://doi.org/10.1016/j.tate.2018.08.008